Italy and Spain are tumbling because one problematic structure (the European Financial Stability Fund, the EFSF) has been conjured up in order to prop up another problematic structure (the eurozone). The result is that both structures are reaching the end of their tether. Enlarging the newer of the two problematic structures (the EFSF) will not make a difference as long it remains… problematic. Italy and Spain, in this sense, are mere casualties of Europe’s determination to retain the EFSF’s structure as is.
Contagion to the eurozone’s core was inevitable because of a potently toxic ingredient deeply buried inside the EFSF’s funding base; not because of its (admittedly puny) size. While all this has been said before (see here and here), the powers-that-be did not listen, the Crisis predictably intensified, and the time has come to say the same things again, perhaps with a little more analysis added for good measure. [Readers averse to a little algebra and geometry are advised to glance at the cobweb-like picture and jump to the conclusion.]
The toxic ratio within the EFSF’s foundations
A eurozone member-state’s debt-to-GDP ratio is the harbinger of ill winds. Especially during a debt crisis, all eyes are on the size of that ratio and on the nation’s growth rate. If the growth rate of member-states with a high debt-to-GDP ratio falls below a certain level, spreads rise and a run on the nation’s bonds is only a matter of time. Once the run is on, the knee-jerk reaction of introducing austerity compresses GDP growth further thus making the problem worse and the run on the member-state’s bonds fiercer. From that moment onwards, the country either defaults or is bailed out by the rest of the eurozone.
This is what happened, for very different reasons, to all the ‘fallen’ eurozone member-states so far (Greece, Ireland and Portugal) and it is the predicament currently felt in Rome and Madrid (with Belgium and France not far behind).
The creation of the EFSF was forced upon Europe by the eruption of the Crisis. Once Germany accepted that the periphery’s fall would signal the eurozone’s end, the search was on for a funding body that would extend loans to the ‘fallen’ albeit without jeopardising the principle of perfectly separable debts (PSDs). PSD means one thing: Each euro of public eurozone debt, including that incurred to bail out the ‘fallen’, must be assigned to one and only one member-state. In practice, it meant that, to remain faithful to the PSD principle, each EFSF-issued bond contained sliced or tranches of debt and each one of these was the liability of a single eurozone member-state ‘donor’.
A byproduct of this toxic structure was that a new, and rather weird, debt-to-GDP ratio entered our lives inconspicuously. Let me explain. Suppose that of the N member-states, F (e.g. 3, as is the case at the moment of writing) have ‘fallen’ out of the money markets and into the EFSF’s bosom. The EFSF must then finance their debts entirely until the Crisis ends. To do so it must seek loan guarantees from the N-F still solvent member-states. It is extremely easy to show that the contribution (as a portion of their GDP) of the N-F solvent member-states to the ‘fallen’ member-states, let’s call it αF (where the subscript indicates the number of ‘fallen’ states that must be supported) equals some newfangled debt-to-GDP ratio: The numerator is the total debt of the ‘fallen’ and the denominator is the total GDP of the still solvent member-states. (See here for a brief proof.) The reason I choose to call αF a toxic ratio is that, with every member-state that ‘falls’, this ratio rises even if GDP and debts remain the same. Moreover, every new casualty boosts the toxic ratio αF and guarantees that yet another member-state will join the rank of the ‘fallen’. And as if this were not enough, nothing can stop this process while everything else remains the same. Including the potential size of the EFSF. The next section explain this dynamic fully.
The explosive EFSF and its poisonous web
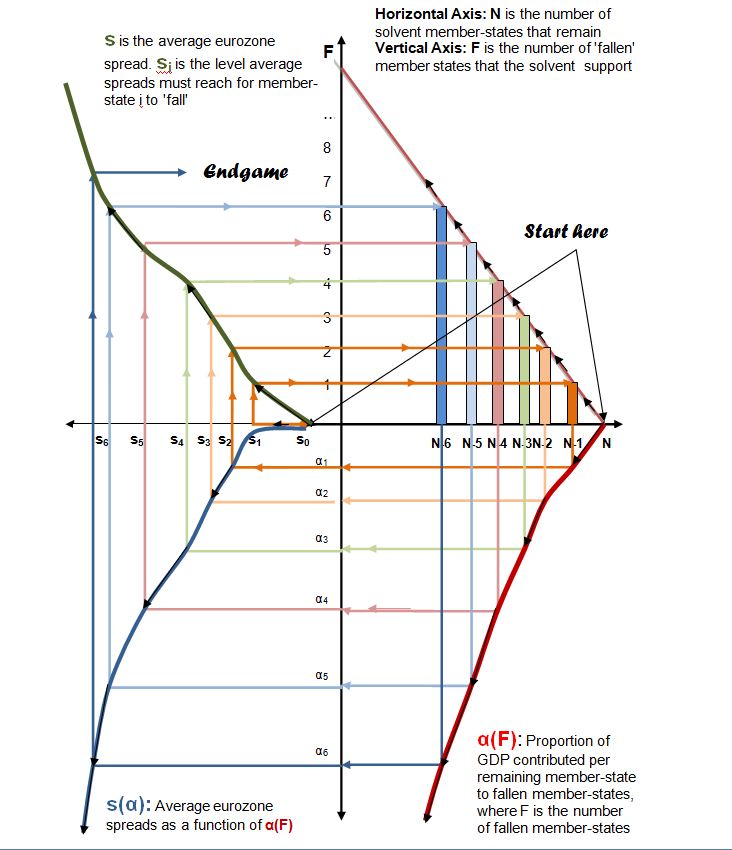This section must be read in conjunction with the adjacent cross diagram (click here for a pdf of the same diagram). The purpose of any cross diagram is to combine four diagrams in one in a manner that makes it easier on the eye to see the interconnections between its parts. To read this particular cross diagram, please note that all axes are positive. For instance, in the bottom right part of the diagram, a downward movement signifies an increasing α(F). Similarly, in the top left part, a leftward movement signifies an increase in s, the eurozone’s average spreads. Having established these simple conventions, it is time to define our four axes.
Top right diagram: The horizontal axis counts the number of solvent member-states. At the outset, i.e. just before the Crisis set in, all N member-states fell in that category. After the first member-state ‘fell’ (i.e. Greece), the number of solvent member states diminished to N-1 (see the horizontal axis) whereas the number of ‘fallen’ states F (see the vertical axis) went up to one. With every member-state that ‘falls’ we climb one notch up the straight line.
Bottom right diagram: Here we find the relationship between the EFSF’s toxic ratio α(F) and the number of ‘fallen’ member-states F. With every new member-state that ‘falls’, we move to the left of the horizontal axis and α(F) rises along the thick red curve. This simply signifies that as more countries fall prey to the Crisis, and require official bail outs from the rest, the remaining solvent countries are facing a rising α(F): Put differently, the ratio of the debts that the solvent must now guarantee over their aggregate GDP increases even if the eurozone’s aggregate debt and aggregate GDP remain the same (indeed, even if the eurozone’s aggregate debt-to-GDP ratio is constant or falling!).
Bottom left diagram: The new axis added here (that runs from the cross diagram’s centre leftward) is the average of the interest rate spreads (i.e. the average difference of member-state interest rates from the lowest interest rate in the eurozone, i.e. Germany’s) facing eurozone’s still solvent states. What happens when α(F) rises in response to the ‘fall’ of a member state that has recently made the awful transition from being an EFSF donor to an EFSF recipient? The simple answer is: Interest rate spreads rise throughout the eurozone. This simple truth is captured here by the blue curve s(α): E.g. When Ireland ‘fell’, α rose and the markets got more jittery about the capacity of Portugal, the new marginal member-state, to shoulder not only its own debt burden but also the added burden of its contribution to the Irish bail out. Markets, in these circumstances, react (naturally) by pushing up the spreads of still solvent nations with a high-ish debt-to-GDP ratio and relatively sluggish growth. Thus, the positive relationship between a(F) and s in this part of our cross diagram.
Top left diagram: Each member-state has a limit beyond which it cannot refinance its existing debt when the interest rates it is called upon to pay reaches a certain threshold level. This is what happened to Greece in May 2010, to Ireland a few months later, to Portugal in the Spring of 2011 and, soon, to Spain and Italy (which will run out of money in September 2011 and February 2012 respectively). In this, final, part of the diagram, the assumption is that pre-crisis average spreads equalled s0. The other values of s, si, depict the level of average spreads facing still solvent member-state i above which it too ‘falls’ and joins the EFSF list of recipient states.
Let us now use this diagram to answer the original questions: Why Italy and Spain? And why is the actual size of the EFSF immaterial? Let us begin our account at the two points that the diagram marks as starting points: In the top right diagram it is point N on the horizontal axis (corresponding to the initial condition when no member-states had yet ‘fallen’) while in the top left diagram it is the average interest rate eurozone spreads level s0 (on the horizontal axis). For reasons that I shall not discuss here, at some point average spreads began to rise sometime toward the end of 2009. When they reached level s1, this triggered the Greek crisis and, after many trials and tribulations, the Greek bail out of May 2010. Once Greece had fallen, the average contribution of each of the remaining member-states, which hitherto equalled zero, rose to level α1. The result was a further increase in average spreads until s reached s2, thus causing Ireland to ‘fall’. [The reader ought to trace the continuous arrow that begins at s0 (see top left diagram), shifts leftwards to s1, then jumps up until F rises from 1 to 2 (i.e. Ireland joins Greece – see top right diagram), then proceeds to the bottom right diagram pushing α to α2 before migrating again to the bottom right diagram where it pushes average spreads to s3, a level that throws Portugal off the cliff etc. etc.
There are two points to note here, before moving to my concluding remarks:
First, the best we can say about our European leaders is that maybe they had hoped that the gradient of curve s(α) would prove less steep and, thus, might have prevented the cobweb-like explosion from occurring. If so, they ought to have known better. For the slope of this curve is not cast in stone but is predicated upon the psychology of the markets. In view of the gross uncertainty at a global level, to bury a toxic ratio, like α(F), in the foundations of your anti-Crisis apparatus (the EFSF) is to ask for trouble.
Secondly, Germany and the rest of the surplus countries were hoping that the loan guarantees that they were offering to the EFSF would never need to turn into actual cash transactions. This would, indeed, be so if the EFSF had a coherent structure: its very institution would have averted speculative games by market traders and German taxpayers would never have had to cough up the euros associated with the loan guarantees to the EFSF. But, with the toxic α(F) inside the EFSF’s foundations, markets recognise the shape of the cross diagram above. And nothing pleases them more than an opportunity to bet against an official’s incredible threat, promise or prediction. If only for this reason, it was insanity personified to imagine that the α(F) curve might turn out slight enough to help contain the contagion. In short, our leaders ought to have known better.
Conclusion
In its attempt to preserve the PSD principle (the idea that all eurozone debts must be separable and attributable to a single member-state) Europe has conjured up a toxic monster by which to resolve an existentialist Crisis. The monster is of course no other than the EFSF and the Crisis is the negative dynamic that threatens credibly to deconstruct the eurozone. Why is the EFSF a monster that is more likely to destroy the eurozone than save it? Because it is an institution that, remarkably, manages, in the middle of a debt crisis, to boost the ratio of the debts that the solvent member-states must guarantee over their aggregate GDP increases even if the eurozone’s aggregate debt and aggregate GDP remain the same (indeed, even if the eurozone’s aggregate debt-to-GDP ratio is constant or falling!).
In this light, the only surprise that Italy and Spain now find themselves inches from an EFSF program is that many were… surprised by this turn of events. The first reason why their surprise is misplaced is that the EFSF’s toxic cobweb-like structure, which is the root cause of the inexorable contagion, remains intact. The second reason is that the latest Greek bail out (see here for my earlier assessment) puts the existing EFSF under an even greater strain therefore increasing its toxicity (increasing further the gradient of the α(F) curve in the preceding cross diagram). In effect, a flimsy, struggling structure has been assigned an even heavier load. Is it any wonder that the Crisis’ poisonous web is spreading its reach further as if in a bid to catch first Spain and then Italy?
Most commentators on the second Greek bail out have commented positively on the easier terms granted to the first and hardest of the ‘fallen. They have also made polite noises about the extension of the EFSF’s remit to include a flexible IMF-like credit line for member-states not yet officially ‘fallen’. “If only”, they add woefully, “the EFSF were extended from the current €440 billion to nearer €2 trillion, the Crisis would end.” By golly are they deluded! What they do not seem to grasp is that, in the EFSF’s case, every new task accelerates the process of the eurozone’s unravelling depicted in the preceding diagram. Speculators will not be deterred by a well funded EFSF as long as the explosive cobweb-like structure of the cross diagram is preserved. While it remains in place, more funds for the EFSF is like more rope for the hangman. Italy and Spain, followed soon by Belgium, will be twisting in the wind however well endowed our leaders decree the EFSF should be.
What could undo the EFSF’s toxicity and puncture a hole through its cobweb-like dynamic? The answer is: the removal of the toxic ratio buried inside. Remove that and all will go swimmingly. But to remove it, Germany and the rest of the surplus nations must give up on the PSD principle (the ‘perfectly separable debts’ dictum) and adopt a genuine eurobond backed up by no guarantees from member states. You see, these guarantees are what create the cobweb-like shape of the current EFSF’s dynamic. The fact that the strongest promises to bail out the second strongest who, in turn, promises to bail out the third strongest, and so on, creates the domino (or, to be more precise, the mountaineering) effect.
To stop this negative dynamic on its tracks Europe needs a common eurobond which represents debt taken out on behalf of the eurozone as a whole with no separate assignments of parts of this debt (at differential interest rates and in the face of differential default probabilities) to different member-states. “But then who will guarantee these eurobonds?” I hear the bond vigilantes ask in earnest. Our suggestion, in the Modest Proposal, is simple: If the ECB issues these eurobonds in order to fund the servicing of tranches of member-states’ existing bonds and, at the same time, opens debit accounts for member-states where the latter will make their long term repayments to the ECB (at interest rates reflecting the ECB-issued eurobonds), then the ECB’s sterling reputation in the global money markets (aided by the common knowledge that, in the final analysis, the ECB has the capacity to monetise debts) will ensure that no further guarantees will be necessary: Investors will flock to buy the ECB’s eurobonds and to fund Europe’s debt relief and recovery (especially under Policy 3 of the Modest Proposal).
The next question that, usually, enters the reader’s mind is: If what you are saying is right, why are Europe’s leaders so committed to the current structure of the EFSF? You may, dear reader, logically conclude that one of two explanations hold: Either my argument here is false or our leaders are irrational. Yet the truth is a little more complicated than that and, therefore, a third explanation may be best: My argument is right and our leaders are rational, albeit in a narrow sense of the word. Put differently, their commitment to the awful EFSF reflects a most peculiar form of rational idiocy. My next post will show what this means and how it is possible that idiocy is reinforced, at a pan-European level, by this narrow form of rationality.
